+) Tích của một số thực \(k\) với một vecto \(\overrightarrow a  \ne \overrightarrow 0 \) là một vecto, kí kiệu là \(k\overrightarrow a .\) +) Vecto \(k\overrightarrow a \) có độ dài bằng \(\left| k \right|\left| {\overrightarrow a } \right|\) và cùng hướng với vecto \(\overrightarrow a \) nếu \(k > 0\), ngược hướng với vecto \(\overrightarrow a \) nếu \(k < 0\)

+) Quy ước: \(0\;\overrightarrow a  = \overrightarrow 0 \) và \(k\;\overrightarrow 0  = \overrightarrow 0 \)

+) Tính chất: Với hai vecto \(\overrightarrow a ,\overrightarrow b \) và hai số thực \(k,t\) ta luôn có:

 \(\begin{array}{l}k(t\overrightarrow a ) = (kt)\;\overrightarrow a \\(k + t)\,\overrightarrow a  = k\overrightarrow a  + t\overrightarrow a \\k(\overrightarrow a  + \overrightarrow b ) = k\overrightarrow a  + k\overrightarrow b ;\quad k(\overrightarrow a  – \overrightarrow b ) = k\overrightarrow a  – k\overrightarrow b \\1\;\overrightarrow a  = \overrightarrow a ;\;\;( – 1)\;\overrightarrow a  =  – \,\overrightarrow a \end{array}\)

Ví dụ: Thực hiện các phép toán vecto sau:

\(\begin{array}{l}
a)5\left( {\overrightarrow u  + \overrightarrow v } \right)\\
b)\left( {x + 2} \right)\overrightarrow a \\
c) – 3\left( {4\overrightarrow e } \right)\\
d)\overrightarrow c  – 2\overrightarrow c 
\end{array}\)

Giải

\(\begin{array}{l}
a)5\left( {\overrightarrow u  + \overrightarrow v } \right) = 5\overrightarrow u  + 5\overrightarrow v \\
b)\left( {x + 2} \right)\overrightarrow a  = x\overrightarrow a  + 2\overrightarrow a \\
c) – 3\left( {4\overrightarrow e } \right) = \left( { – 3.4} \right)\overrightarrow e  =  – 12\overrightarrow e \\
d)\overrightarrow c  – 2\overrightarrow c  = \left( {1 – 2} \right)\overrightarrow c  = \left( { – 1} \right)\overrightarrow c  =  – \overrightarrow c 
\end{array}\)

Hai vecto \(\overrightarrow a \) và \(\overrightarrow b \) (\(\overrightarrow b \) khác \(\overrightarrow 0 \))cùng phương khi và chỉ khi tồn tại \(k\) sao cho \(\overrightarrow a  = k\overrightarrow b .\)

Nhận xét: Ba điểm phân biệt A, B, C thẳng hàng \( \Leftrightarrow \overrightarrow {AB}  = k\overrightarrow {AC} .\)

Chú ý: Cho hai vecto \(\overrightarrow a \) và \(\overrightarrow b \) không cùng phương. Với mỗi vecto \(\overrightarrow c \) luôn tồn tại duy nhất cặp số thực \((m;n)\) sao cho \(\overrightarrow c  = m\,\overrightarrow a  + n\,\overrightarrow b \)

Ví dụ: Cho tam giác ABC có trung tuyến AM. Gọi I là trung điểm của AM và K là điểm trên cạnh AC sao cho \(AK = \frac{1}{3}AC\). 

a) Tỉnh \(\overrightarrow {BI} \) theo \(\overrightarrow {BA} \), \(\overrightarrow {BC} \).

b)Tính \(\overrightarrow {BK} \) theo \(\overrightarrow {BA} \), \(\overrightarrow {BC} \).

c) Chứng minh ba điểm B, I, K thẳng hàng.

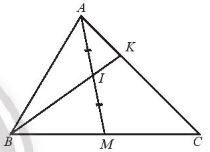
Giải

a) \(\overrightarrow {BI}  = \overrightarrow {BA}  + \overrightarrow {AI}  = \overrightarrow {BA}  + \frac{1}{2}\overrightarrow {AM}  = \overrightarrow {BA}  + \frac{1}{2}\left( {\overrightarrow {BM}  – \overrightarrow {BA} } \right) = \frac{1}{2}\overrightarrow {BA}  + \frac{1}{4}\overrightarrow {BC} \) (1)

b) \(\overrightarrow {BK}  = \overrightarrow {BA}  + \overrightarrow {AK}  = \overrightarrow {BA}  + \frac{1}{3}\overrightarrow {AC}  = \overrightarrow {BA}  + \frac{1}{3}\left( {\overrightarrow {BC}  – \overrightarrow {BA} } \right) = \frac{2}{3}\overrightarrow {BA}  + \frac{1}{3}\overrightarrow {BC} \) (2)

c) Ta có:

\(\begin{array}{l}
\left( 1 \right) \Rightarrow 4\overrightarrow {BI}  = 2\overrightarrow {BA}  + \overrightarrow {BC} \\
\left( 2 \right) \Rightarrow 3\overrightarrow {BK}  = 2\overrightarrow {BA}  + \overrightarrow {BC} 
\end{array}\)

Nên \(\overrightarrow {BI}  = \frac{3}{4}\overrightarrow {BK} \) (3)

Từ (3) suy ra ba điểm B, I, K thẳng hàng